Level curves allow to visualize functions of two variables f(x,y) Example For f(x,y) = x2− y2 the set x2− y2= 0 is the union of the lines x = y and x = −y The set x2− y2= 1 consists of two hyperbola with with their "noses" at the point (−1,0) and (1,0)TwoDimensional Calculus (11) Chapter 2 Differentiation 8 Level curves and the implicit function theorem Let f(x, y) be continuously differentiable in a domain D and let (x 0, y 0) be any point in DThe equation f(x, y) = f(x 0, y 0) defines a level curve through the point (x 0, y 0)Let us assume for the moment that this level curve is the implicit form of a regular curve C, at leastLearn on any device · Realworld projects · 24/7 Customer Support · 150 University Partners
Calculus Iii Functions Of Several Variables
Level curves of utility function
Level curves of utility function-May 26, · The level curves of the function \(z = f\left( {x,y} \right)\) are two dimensional curves we get by setting \(z = k\), where \(k\) is any number So the equations of the level curves are \(f\left( {x,y} \right) = k\) Note that sometimes the equation will be in the form \(f\left( {x,y,z} \right) = 0\) and in these cases the equations of the level curves are \(f\left( {x,y,k} \right) = 0\)Describe the level curves of the function z = 12 4x 3y, c = 0, 2, 4, 6, 8, 10 O The level curves are parallel lines The level curves are hyperbolas The level curves are noncircular ellipses The level curves are circles O The level curves are parabolas
1
Level Curve Grapher Level Curve Grapher Enter a function f (x,y) Enter a value of c Enter a value of c Enter a value of c Enter a value of cCourseraorg has been visited by 10K users in the past monthSelect a function from the dropdown menu or type your own function in the text box below and click "Enter" to plot it Click the radio buttons to view either a level curve or a cross section Use the slider to change the value of the related constant k, c, or d Click "Reset" to reset both plots
A level curve of a function f (x, y) is the curve of points (x, y) where f (x, y) is some constant value A level curve is simply a cross section of the graph of z = f (x, y) taken at a constant value, say z = c A function has many level curves, as one obtains a different level curve for each value of c in the range of f (x, y)Level Curves We are given a function two variables {eq}f(x,y) {/eq} The level curves are represent the set of points in the xy plane where the function assumes a constant valueDefine the function by fx_,y_ = (x^2 4 y^2) Exp1 x^2 y^2
Image transcriptions Given, fx}y}= 1L54s24_}r1 —5 We have to determine the level curves of the function We know that level curves anj,F function f Ly is given by" f 1 y) = k , therefore 15;2 4y1—5=k I 4y1=k5 2 2 x y _ k5 k5 _1 15 4 If we change the values of l we get the different ellipses because this is an equation of ellipse with major axis as y axisMar 30, 16 · A level curve of a function of two variables is completely analogous to a contour line on a topographical map (a) A topographical map of Devil's Tower, Wyoming Lines that are close together indicate very steep terrain (b) A perspective photo of Devil's Tower shows just how steep its sides are Notice the top of the tower has the sameThe level curves are in the range of the function The level curves are on the surface The level curves can also be thought of as the intersection of the plane with the surface We often mark the function value on the corresponding level set If we choose function values which have a constant difference, then level curves are close together
Contour Maps In Matlab
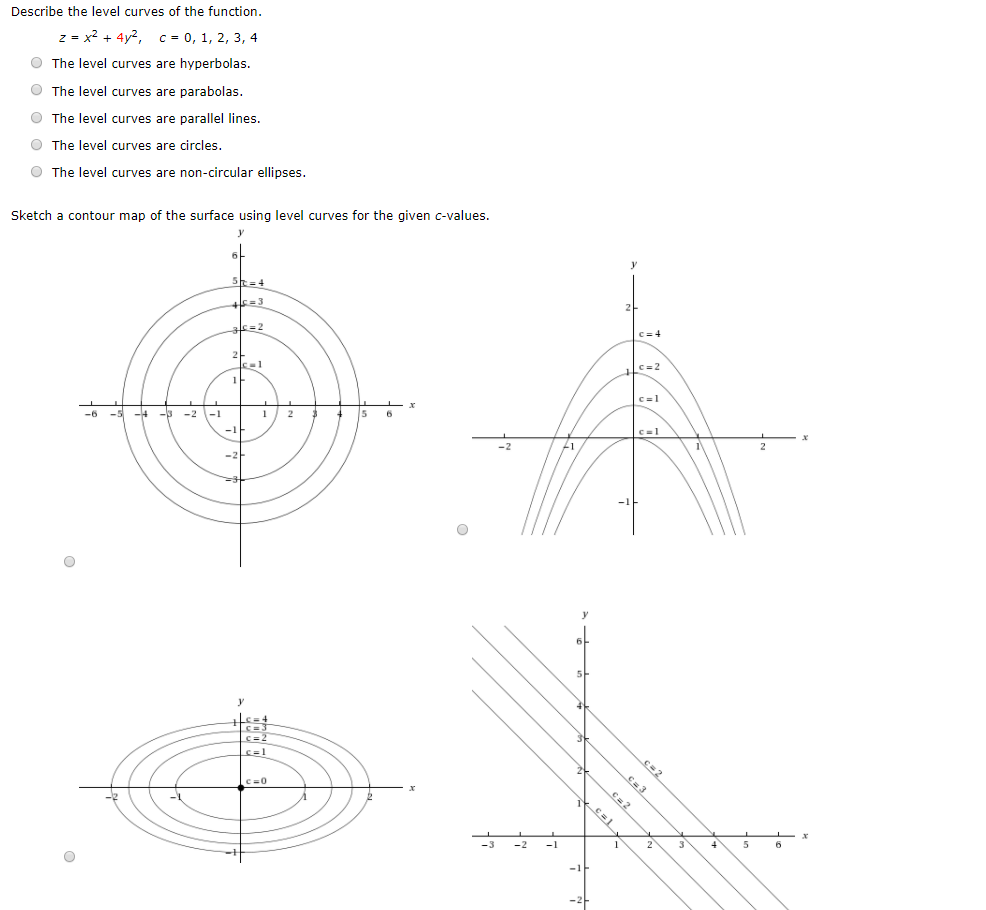
Describe The Level Curves Of The Function Z X2 Chegg Com
Get the free "Level Curves" widget for your website, blog, Wordpress, Blogger, or iGoogle Find more Mathematics widgets in WolframAlphaLevel Curves In mathematics, a level set of a realvalued function f of n real variables is a set where the function takes on a given constant value cLevel Curves Def If f is a function of two variables with domain D, then the graph of f is {(x,y,z) ∈ R3 z = f(x,y) } for (x,y) ∈ D Def The level curves of a function f(x,y)are the curves in the plane with equations f(x,y)= kwhere is a constant in the range of f The contour curves are the corresponding curves on the surface, the
Use The Level Curves Of The Function To Determine If Each Partial Derivative At The Point P Is Positive Negative Or Zero Mathematics Stack Exchange
Www Math Uci Edu Remote Teaching Lecture Notes Of Hamed Math 2d lecture notes Lecture note 14 1 Pdf
The Gradient and the Level Curve Our text does not show this, but the fact that the gradient is orthogonal to the level curve comes up again and again, and in fact, the text proves a more complicated version in three dimensions (the gradient is orthogonal to the level surface) It is important, so we go through a proof and an exampleGradients, Normals, Level Curves Objectives In this lab you will demonstrate the relationship between the gradients and level curves of functions The Gradient as a Vector Operator The gradient of a function, is a vector whose components are the partials of the original function;Jul 09, 19 · How to Find the Level Curves of a Function Calculus 3 How to Find the Level Curves of a Function Calculus 3
Find The Level Curve Through The Point On The Gradient Physics Forums
Level Set Wikipedia
Where f(x;y)=x3 y3 −3xyConsequently,Equation 6 is the level curve f(x;y) = 0 of the function f(x;y)=x3y3−3xy You can plot a single level curve of a function by using Matlab's contourcommand in the form contour(x,y,z,c c) The following commands should produce an image similar to that in Figure 7 Note how a ner meshLevel Curves and Surfaces The graph of a function of two variables is a surface in space Pieces of graphs can be plotted with Maple using the command plot3dFor example, to plot the portion of the graph of the function f(x,y)=x 2 y 2 corresponding to x between 2 and 2 and y between 2 and 2, type > with (plots);Jan 21, · A level curve of a function f(x,y) is the curve of points (x,y) where f(x,y) is some constant value A level curve is simply a cross section of the graph of z=f(x,y) taken at a constant value, say z=c A function has many level curves, as one obtains a different level curve for each value of c in the range of f(x,y)
Www Jstor Org Stable
1
Level curves, contour curves Definition The level curves of a function f D ⊂ R2 → R ⊂ R are the curves in the domain D ⊂ R2 of f solutions of the equation f (x,y) = k, where k ∈ R is a constant in the range of f The contour curves of function f are the curves in R3 given by theSep 19, 17 · If you take a perfectly horizontal sheet or plane that's parallel to the xyplane, and you use that to slice through your threedimensional figure, then what you get at the intersection of the figure and the plane is a twodimensional curve What we want to be able to do is slice through the figure at all different heights in order to get what we call the "level curves" of a functionJun 07, 15 · By letting Z equal to some constant 'c' we get a single level curve I would like to obtain an expression of the resulting function of the form y=f(x) to be able to study other properties of it Basic Example 1 Easy game Let's consider the problem of plotting level curves of z=x^2y^2100 for x,y10;10 and z=1
Level Set Examples Math Insight
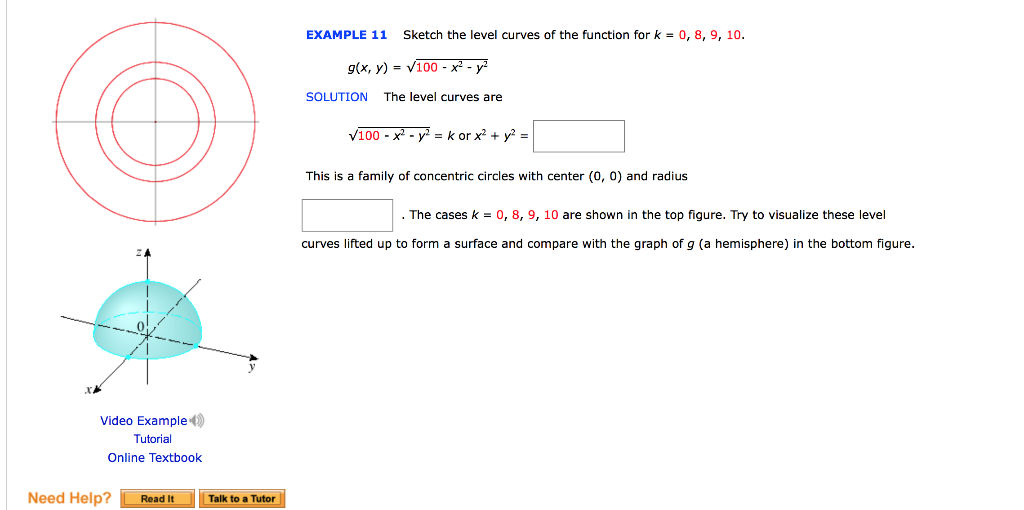
Solved Example 11 Sketch The Level Curves Of The Function Chegg Com
Level Curves So far we have two methods for visualizing functions arrow diagrams and graphs A third method, borrowed from mapmakers, is a contour map on which points of constant elevation are joined to form contour curves, or level curves A level curve f (x, y) = k is the set of all points in the domain of f at which f takes on a given value kSo level curves, level curves for the function z equals x squared plus y squared, these are just circles in the xyplane And if we're being careful and if we take the convention that our level curves are evenly spaced in the zplane, then these are going to get closer and closer together, and we'll see in a minute where that's coming fromSketch the level curves for c=1,0,1,2 of the given function The plane in Exercise 2
Riemann Zeta Function Graphics
Http Www Math Drexel Edu Classes Calculus Resources Math0hw Homework13 1 Ans Pdf
The level curve of this function for the value 2 is empty (there are no values of (x, y) such that f(x, y) = 2) and the level curve for the value 1 is the set all all points (x, y) In less extreme examples, some but not all level curves are setsAdBuild your Career in Data Science, Web Development, Marketing & More Flexible, Online Learning at Your Own Pace Invest 23 Hours A Week & Advance Your CareerLevel surfaces For a function $w=f(x,\,y,\,z) \, U \,\subseteq\, {\mathbb R}^3 \to {\mathbb R}$ the level surface of value $c$ is the surface $S$ in $U \subseteq
Level Sets Ximera
Math Msu Edu Robertbe Lb2sp13 S4 Pdf
Relief Functions and Level Curves Purpose The purpose of this lab is to introduce you to plots of relief functions and level curves on surfaces Several Maple procedures will be introduced to help with visualization Background In this lab we will consider the case of a surface defined explicitly by an equation of the form z = f(x, y)Level Curves For a general function z = f ( x, y), slicing horizontally is a particularly important idea Level curves for a function z = f ( x, y) D ⊆ R 2 → R the level curve of value c is the curve C in D ⊆ R 2 on which f C = cThen the curves obtained by the intersections of the planes $z = k$, $k \in \mathbb{R}$ with the graph of $f$ are called the Level Curves of $f$ From the definition of a level curve above, we see that a level curve is simply a curve of intersection between any plane parallel to the $xy$ axis and the surface generated by the function $z = f(x, y)$
Level Curves Example 1
Answer In Differential Geometry Topology For Shweta 1350
Jun 21, · Consider the function f (x, y) = (x − 1) 2 y e x 3 y Setting it equal to zero, we get x = 1 or y = 0 According to my understanding, these two lines should be the level curves However, if I plot the function using a 3D plotter (GeoGebra in my case), it only seems to show y = 0 as the level curve (the black line in the figure)Level Curves Author Kristen Beck Topic Functions This worksheet illustrates the level curves of a function of two variables You may enter any function which is a polynomial in both andLet us first recall briefly how level curves (isolines) work as we translate from three dimensions into a twodimensional graph We start with a threedimensional function And we slice that function with a plane along specific values of one of the variables (typically the zdirection), and then project that intersection onto the two
Contour Maps In Matlab
Level Curves Calculus
Level Curves and Contour Plots Level curves and contour plots are another way of visualizing functions of two variables If you have seen a topographic map then you have seen a contour plot Example To illustrate this we first draw the graph of z = x2 y2 On this graph we draw contours, which are curves at a fixed height z = constantA level curve can be drawn for function of two variable ,for function of three variable we have level surface A level curve of a function is curve of points where function have constant values,level curve is simply a cross section of graph of fNov 10, · Returning to the function \(g(x,y)=\sqrt{9−x^2−y^2}\), we can determine the level curves of this function The range of \(g\) is the closed interval \(0,3\) First, we choose any number in this closed interval—say, \(c=2\) The level curve corresponding to \(c=2\) is described by the equation \ \sqrt{9−x^2−y^2}=2\
Level Surfaces
13 1
Jul 01, 21 · Find the equations of level curves of the function z= =f (x, y) = 369x² 4y2, and sketch the graphs of these level curves 7 Use implicit differentiation to find Oz дх дz and if x² y2 z² – 2xyz = 5 This question hasn't been solved yetOne level curve is defined as f (x, y) = c 1, the other as f (x, y) = c 2 If c 1 ≠ c 2 (else they are the same curve), if there exists a point on both level curves, that would mean f (x, y) = c 1 and f (x, y) = c 2, meaning c 1 = f (x, y) = c 2 which cannot be trueFeb 25, 04 · A level curve of a function f (x,y) is a set of points (x,y) in the plane such that f (x,y)=c for a fixed value c Example 5 The level curves of f (x,y) = x 2 y 2 are curves of the form x 2 y 2 =c for different choices of c These are circles of radius square root of c
Mysite Science Uottawa Ca ionne Teaching Help Centre Level Curve Pdf
Evpuhoqhnzjyfm
Describe the level curves of the function Sketch the level curves for the given c values f (x, y) = e x y / 2, c = 2, 3, 4, 1 2, 1 3, 1 4Definition The level curves of a function f of two variables are the curves with equations f (x,y) = k, where k is a constant (in the range of f ) A level curve f (x,y) = k is the set of all points in the domain of f at which f takes on a given value k In other words, it shows where the graph of f has height k1 Level Surfaces 2 If one of the Arguments is time we can animate ie w = f(x,y,t) Level Surfaces Given w = f(x,y,z) then a level surface is obtained by considering w = c = f(x,y,z) The interpretation being that on a level surface f has the same value at every pt For example f could represent the temperature at each pt in 3space
Http Www Personal Psu Edu Sxj937 Math111 Lecture 11 Pdf
Level Curves Of Functions Of Two Variables Youtube
Chapter 14 Partial Derivatives Chapter 14 Partial Derivatives Ppt Download
1 Sketch A Few Of The Level Curves Of The Function F X Y Surface Z Homeworklib
The Field Of The Condenser And The Level Curves Of The Function U For Download Scientific Diagram
Level Curves Geogebra
Http Math Wustl Edu Beheshti 14 1 Sol Pdf
The Figure Shows Level Curves Of A Function F In The Square R 0 6 Times 0 6 Use The Midpoint Rule With M N 2 To Estimate
Help With Partial Derivatives On A Level Curve Calculus
Level Sets Math Insight
Level Curves
Problem On Gradient Directional Derivative And Level Curves Leading Lesson
Calculus Iii Functions Of Several Variables
Www Math Uci Edu Remote Teaching Lecture Notes Of Hamed Math 2d lecture notes Lecture note 14 1 Pdf
Section 13 1 Level Curves Youtube
Oneclass Use The Level Curves Of The Function Z F X Y To Decide The Sign Positive Negative Or
Hgm4 14 Review 71 Gradients Etc Pg Query
0 3 Visualizing Functions Of Several Variables
Answered Describe The Level Curves Of The Bartleby
Math 2110 Section 13 1 Level Curves And Level Surfaces Youtube
Ppt Multivariable Functions Of Several Their Derivatives Powerpoint Presentation Id
Sketch The Level Curves Of The Function G X Y Chegg Com
Level Curves Geogebra
Matlab How Can I Get The Expression Of The Level Curves Of A Function Stack Overflow
Sketch The Level Curves Of The Function F X Y X Y 4 Mathematics Stack Exchange
Calculus 11 1 Functions Of Several Variables Flashcards Quizlet
Level Curves Curves Level Stvincent Glogster Edu Interactive Multimedia Posters
Describe The Level Curves Of The Function Sketch The Level Curves For The Given Values Of C F X Y X 2 2y 2 C 0 1 2 3 4 Study Com
Level Sets Ximera
Get Answer Figure 18 61 Shows Level Curves Of The Function F X Y A Transtutors
Calculus Iii Functions Of Several Variables
13 1 Functions Of Several Variables Mathematics Libretexts
Describe The Level Curves Of The Function Z 8 2x 7y Chegg Com
Level Curves And Implicit Differentiation Studocu
Graphs And Level Curves
Mcmaster University Math Test 1m03 12 Level Curves Of Function Topic Play
0 3 Visualizing Functions Of Several Variables
2
Vb Helper Howto Draw Contour Plots Level Curves For A Function With Known Derivatives
1
Lecture Notes Chapter 1 1 Part2 Contourlines Level Curves And 3d Graphs Pdf
Gate Ese Level Curves And Surface Of A Function In Hindi Offered By Unacademy
Math 27 Winter 12 Assignment 2 1 Sketch The Level Curves Of
Contour2d Level Curves Of A Surface On A 2d Plot
Answered The Figure Below Shows The Level Curves Bartleby
Draw Level Curves For Functions Of Two Variables In C C Helper
1
Level Curves
Solved The Figure Shows Level Curves Of A Functio
Using Polar Coordinates Describe The Level Curves Of The Function Defined By F X Y 2xy X 2 Y 2 Hspace 0 2cm Text If Hspace 0 2cm X Y Neq 0 0 Hspace 0 2cm Text And Hspace 0 2cm F 0 0 0 Homework Help And Answers
Relief Functions And Level Curves
Calculus Iii Functions Of Several Variables
13 1
The Level Curves Of The Function U For The Condenser In Example 6 3 For Download Scientific Diagram
2
Level Curves Geogebra
Draw A Contour Map Of The Function Showing Several Level Curves F X Y Y 8x 2 Brainly Com
Level Set Wikipedia
Level Curves
Level Sets Math Insight
14 Partial Derivatives Ppt Video Online Download
Note On The Curvature Of Orthogonal Trajectories Of Level Curves Of Green S Function Pnas
Sketching Level Curves Of A Function For Given Values Mathematics Stack Exchange
1
Level Curves Of The Error Function Download Scientific Diagram
How To Sketch Level Curves Youtube
Multivariable Functions Level Curves And Partial Derivatives
Level Sets Math Insight
Contour Map Of The Function Showing Several Level Curves Physics Forums
Solved The Figure Shows Level Curves Of A Functio
Graphs And Level Curves
Calculus 11 1 Functions Of Several Variables Flashcards Quizlet
Solved Draw A Contour Map Of The Function Showing Several Level Curves F X Y Sqrt X Y
Draw The Level Curve Of The Function F X Y X Y Containing The Point 3 3 Study Com
28 36 38 40 41 15 1 Graphs And Level Curves 927 A Figure 15 18 Section 15 1 Homeworklib
Gradients And Level Curves
Find Two Level Curves Of The Function F X Y X Y X Y X Y And Sketch Them Socratic
Relief Functions And Level Curves
Level Curves
Lagrange Multipliers
Www3 Nd Edu Taylor Math550 Images Currentexamsolutions Exam2f16 Pdf
Answered Sketch The Level Curves Of The Function Bartleby
0 件のコメント:
コメントを投稿